Find The Volume Of A Sphere With A Radius Of 6 Cm

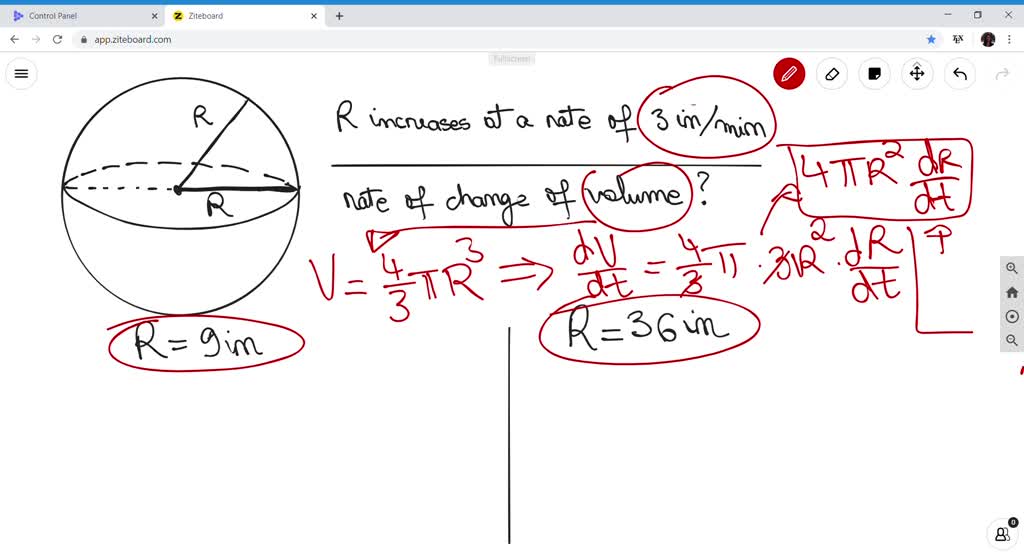
The unit of volume of a sphere is given as the 3. The metric units of volume are cubic meters or cubic centimeters while the USCS units of volume are, cubic inches or cubic feet. The volume of sphere depends on the radius of the sphere, hence changing it changes the volume of the sphere.

There are two types of spheres, solid sphere, and hollow sphere. The volume of both types of spheres is different. We will learn in the following sections about their volumes. For example, the space that a substance or 3D shape occupies or contains. Volume is often quantified numerically using the SI derived unit, the cubic metre. Volumes of some simple shapes, such as regular, straight-edged, and circular shapes can be easily calculated using arithmetic formulas.

Volumes of complicated shapes can be calculated with integral calculus if a formula exists for the shape's boundary. One-dimensional figures and two-dimensional shapes are assigned zero volume in the three-dimensional space. Calculating volume and surface area of sphere play an important role in mathematics and real life as well. Formulas for volume & surface area of sphere can be used to explore many other formulas and mathematical equations. Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities. Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage.

It is important to be able to calculate the volume and surface area of these solids. Now the question becomes calculating the volume of the bicylinder . It is also very difficult, so add a cube packing the bicylinder .

Now when the plane intersects the cube, it forms another larger square. The extra area in the large square , is the same as 4 small squares . Moving through the whole bicylinder generates a total of 8 pyramids. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cn and its height is 180 cm. These solids differ from prisms in that they do not have uniform cross sections.

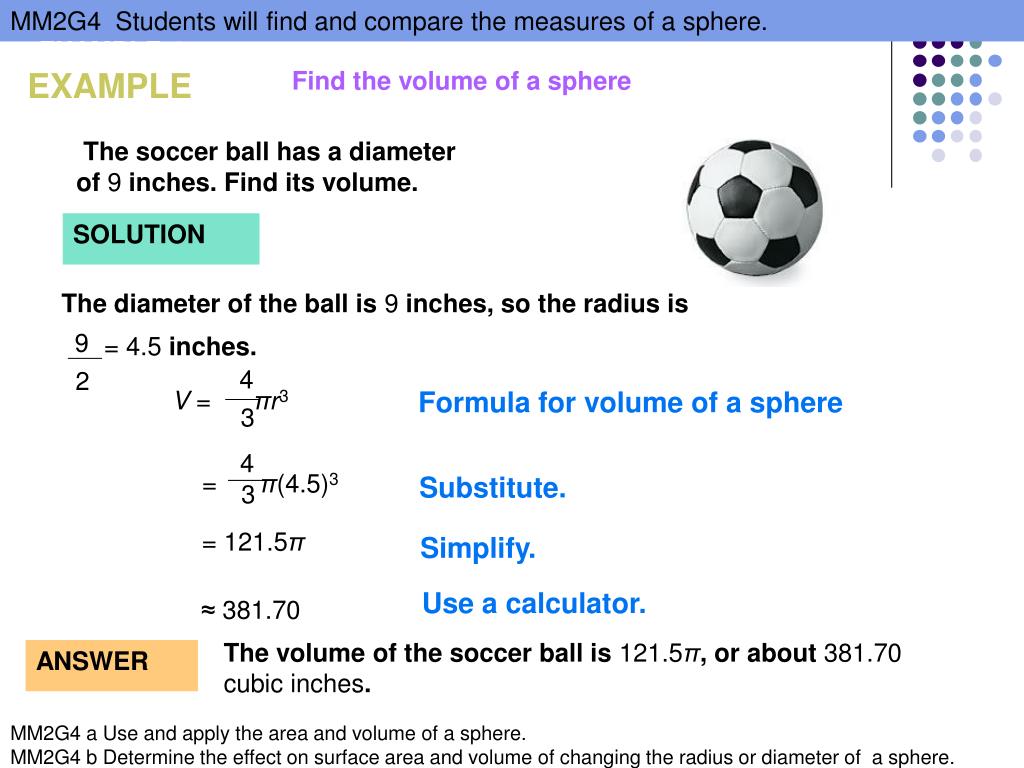
A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the center of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below. If you ever wondered what's the volume of the Earth, soccer ball or a helium balloon, our sphere volume calculator is here for you. It can help to calculate the volume of the sphere, given the radius or the circumference. Also, thanks to this calculator you can determine the spherical cap volume or hemisphere volume.

Volume is the amount of total space on the interior of the solid. Knowing the definition of volume, we can now focus on the formulas for volume of common geometric solids. Using these formulas manually won't be difficult, but for fast, accurate results every time, use the volume calculator. The volume of sphere is the capacity it has.

It is the space occupied by the sphere. The volume of sphere is measured in cubic units, such as m3, cm3, in3, etc. The shape of the sphere is round and three-dimensional. It has three axes as x-axis, y-axis and z-axis which defines its shape. All the things like football and basketball are examples of the sphere which have volume.

In this lesson, you'll learn how to find the volume of a sphere with a radius of 4 inches. A sphere is a 3-dimensional round object. The volume is how space is inside the sphere. When you want to find out how much space is inside such a sphere, you'll follow these steps to calculate the volume of the sphere. This online calculator will calculate the 3 unknown values of a sphere given any 1 known variable including radius r, surface area A, volume V and circumference C.

It will also give the answers for volume, surface area and circumference in terms of PI π. A sphere is a set of points in three dimensional space that are located at an equal distance r from a given point . In this example you need to calculate the volume of a very long, thin cylinder, that forms the inside of the pipe. The area of one end can be calculated using the formula for the area of a circle πr2. The diameter is 2cm, so the radius is 1cm. The area is therefore π × 12, which is 3.14cm2.

A sphere is a round shape solid object in three-dimensional space. It can be defined as the set of points that are all at the same distance from a given point . The perfect example of the sphere is the globe and ball.

The following figure shows a sphere shape whose radius is r, and the diameter is d. There is another special formula for finding the volume of a sphere. The volume is how much space takes up the inside of a sphere.

The answer to a volume question is always in cubic units. For any other value for the length of the radius of a sphere, just supply a positive real number and click on the GENERATE WORK button. They can use these methods in order to determine the surface area and volume of parts of a sphere. A circle can be drawn on a paper but a sphere can't be drawn on a piece of paper. This is because Circle is a two-dimensional figure whereas a sphere is a three-dimensional object, example- Ball, Earth, etc. A Sphere is a 3D figure whose all the points lie in the space.

All the points on the surface of a sphere are equidistant from its centre. This distance from the surface to the centre is called the radius of the sphere. A glass dome for a lighting fixture is in the shape of a hemisphere. The circumference of the great circle of the hemisphere is 12π inches. Which statements about the hemisphere are true? The total surface area is 108π square inches.

The total surface area is 144π square inches. The total surface area is 432π square inches. The total surface area is 36π square inches. If the baseball has a surface area of 9π, then I can set that equal to the formula 4πr2 and solve for r. The radius is 3/2 inches, so I double that to find the diameter.

The diameter of the ball is 3 inches, which is greater than the allowed range of diameters. A sphere is a three-dimensional solid with no base, no edge, no face and no vertex. Sphere is a round body with all points on its surface equidistant from the center. The volume of a sphere is measured in cubic units. A sphere is a three-dimensional solid with no face, no edge, no base and no vertex. It is a round body with all points on its surface equidistant from the center.

The volume of a sphere is the measurement of the space it can occupy. A sphere is a three-dimensional shape that has no edges or vertices. In this short lesson, we will learn to find the volume of a sphere, deduce the formula of volume of a sphere and learn to apply the formulas as well. Once you understand this chapter you will learn to solve problems on the volume of the sphere. In this calculation you can calculate the volume of a sphere with a number of given input values, such as radius, diameter, circumference. You also have a number of different input units and can choose output unit according to your likings.

A set of points in a space equally distanced from a given point $O$ is a sphere. The point $O$ is called the center of the sphere. The distance from the center of a sphere to any point on the sphere is called the radius of this sphere.

A radius of a sphere must be a positive real number. The segment connecting two points on the sphere and passing through the center is called a diameter of the sphere. All radii of the sphere are congruent to each other. A sphere can be obtained by rotating a semicircle about the diameter. Two spheres of the same radius are congruent. The following video shows how to solve problems involving the formulas for the surface area and volume of spheres.

Q.9.A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as show in Fig. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article. Q.5.A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube. Determine the surface area of the remaining solid. The volume of a sphere is the three-dimensional space occupied by a sphere. This volume depends on the radius of the sphere (i.e, the distance of any point on the surface of the sphere from its centre).

If we take the cross-section of the sphere then the radius can be calculated by reducing the length of the diameter to its half. Or we can also say that the radius is half of the diameter. The volume of sphere formula can be given for a solid as well as the hollow sphere. Calculate the volume of all common geometrical shapes, such as cubes, spheres, pyramids, cones etc. It turns out that the volume formula works just the same for these. You must however use the perpendicular height in the formula.

This is the vertical line to left in the figure above. To illustrate this, check 'Freeze height'. As you drag the top of the cylinder left and right, watch the volume calculation and note that the volume never changes. This statement is not at all obvious or elementary.

"A sphere's volume is two cones of equal height and radius to that of the sphere's". The assertion about the cone and the cylinder is a little easier to prove, but it too is not obvious. So you have not really provided an answer to this to year old question.

I think the accepted answer is closest to what you have in mind. If you want to help here I think you should pay attention to new questions that don't yet have answers. This concept can be of significance in geometry, to find the volume & surface area of sphere and its parts. Real life problems on volume & surface area of sphere are very common, so this concept can be of great importance of solving problems.

The following figure gives the formula for the volume of sphere. Scroll down the page for examples and solutions. Just split the solid up into smaller parts until you reach only polyhedrons that you can work with easily. Q.1.A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.

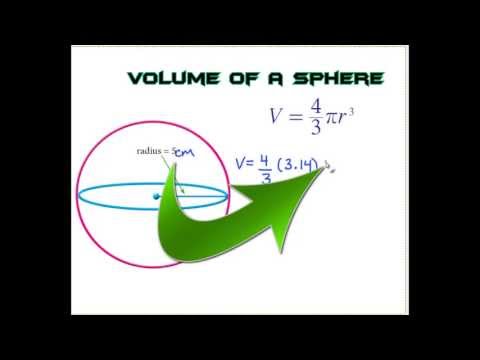
The volume of a sphere is the amount of space occupied by it. For a hollow sphere like a football, the volume can be viewed as the number of cubic units required to fill up the sphere. Let us take an example to learn how to calculate the volume of sphere using its formula. The volume of a 3 -dimensional solid is the amount of space it occupies. Be sure that all of the measurements are in the same unit before computing the volume.

7Given a solid sphere of radius R, remove a cylinder whose central axis goes through the center of the sphere. Suppose you have two solid figures lined up next to each other, each fitting between the same two parallel planes. (E.g., two stacks of pennies lying on the table, of the same height). Then, consider cutting the two solids by a plane parallel to the given two and in between them. If the cross-sectional area thus formed is the same for each of the solids for any such plane, the volumes of the solids are the same.

The formula for the volume of a sphere is 4/3 times pi times the radius cubed. Cubing a number means multiplying it by itself three times, in this case, the radius times the radius times the radius. There are many applications in real life where the volume calculator is useful. One such instance is in road or pavement construction where slabs of concrete must be built. Generally concrete slabs are rectangular solids, so the rectangular prism calculator can be used. In the imperial/English system the equivalent measurements are fluid ounces, pints, quarts and gallons, which are not easily translated into cubic feet.

It is therefore best to stick to either liquid or solid volume units. Q.6.A medicine capsule is in the shape of a cylinder with two hemispheres stuck to each of its ends (see Fig.). The length of the entire capsule is 14 mm and the diameter of the capsule is 5 mm. Q.3.A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius. The total height of the toy is 15.5 cm.

Find the total surface area of the toy. Q.2.A vessel is in the form of a hollow hemisphere mounted by a hollow cylinder. The diameter of the hemisphere is 14 cm and the total height of the vessel is 13 cm. Find the inner surface area of the vessel. Half of the Sphere is called Hemisphere.